Nonsingular Linear Transformation Matrix
A non-singular matrix is a square one whose determinant is not zero. If Tis invertible then it is nonsingular but if Tis nonsingular then it is not necessarily invertible.
Q1 Let B Be A Skew Symmetric Matrix With Real Chegg Com
Since the n dimensional parallelopiped formed by.

Nonsingular linear transformation matrix. Singular and nonsingular transformations. There can only be one inverse as Theorem 13 shows. An invertible linear transformation TV-W is a map between vector spaces V and W with an inverse map which is also a linear transformation.
Let H be a nonsingular linear transformation. A linear transformation is nonsingular when its nullity is zero that is when its kernel is the trivial subspace f0g. An matrix is called nonsingular if the only solution of the equation is the zero vector.
It follows that a non-singular square matrix of n nhas a rank of n. Otherwise is called singular. The derivative of the matrix exponential is given by the formula.
If A has an inverse matrix A1 then eAeA I. I know that every nonsingular square matrix is invertible. If it is singular matrix then A0.
If A H M H 1 then etA H etM H 1. The rows of A are linearly dependent O c. The next theorem pulls a lot of big ideas together.
A linear transformation Y AX is called nonsingular if the images of distinct vectors X i are distinct vectors Y i. N A 0 N A 0. One more thing is to be noted here if A-1 exist then matrix A must be non singular ie.
True If A is an n x n nonsingular matrix then Select one. The author might be calling T nonsingular because it comes with a well-defined inverse at least on the range of T. Suppose that A A is a square matrix.
Suppose that u 2 U lies in the kernel of S T. It follows immediately that T is injective since if T v 1 T v 2 then T v 1 T v 2 T v 1 v 2 0 and hence v 1 v 2 0 or equivalently v 1 v 2. The columns of A are linearly dependent O.
When T is given by matrix multiplication ie TvAv then T is invertible iff A is a nonsingular matrix. A If A and B are n n nonsingular matrix then the product AB is also nonsingular. Just as we can use the inverse of the coefficient matrix to find the unique solution of any linear system with a nonsingular coefficient matrix Theorem SNCM we can use the inverse of the linear transformation to construct the unique element of any pre-image proof of Theorem ILTIS.
W be the linear transformations in question. Just like the formula for a 2 2 matrix the general formula includes the coefficient 1 det A and a matrix related to the original matrix. A must be non zero.
If A does not have an inverse A is called singular. We must show that u 0. But Tcolonmathbb R2tomathbb R3such that Txyxyx-2y3xyis nonsingular but inverse does not exist.
If A be a non singular matrix then A is not equal to 0. Theorem NMTNS Nonsingular Matrices have Trivial Null Spaces. So every nonsingular linear transformation should also invertible.
A linear transformation Y AX is nonsingular if and only if A the matrix of the transformation is nonsingular. This means that there is a linear combination of its columns not all of whose coefficients are 0 which sums to the 0 vector. A nonsingular linear transformation carries linearly.
Since u 2 kerS T we see that as elements of W 0 S Tu STu. A 1 1 det A d b c a This formula is a special case of a general formula for the inverse of a nonsingular square matrix. The rank of a matrix A is equal to the order of the largest non-singular submatrix of A.
A Show that if and are nonsingular matrices then the product is also nonsingular. Suppose T v 0 implies v 0. N A 0 O b.
An n n matrix A is called nonsingular or invertible if there exists an n n matrix B such that. EmAenA emnA where mn are arbitrary real or complex numbers. B Show that if is nonsingular then the column vectors of are linearly independent.
Therefore we can unambiguously define T 1 on the range of T. A matrix B such that AB BA I is called an inverse of A. Thus a non-singular matrix is also known as a full rank matrix.
Using the definition of a nonsingular matrix prove the following statements. If determinant of scalar value of a square matrix is non zero then it is called a non singular matrix. D dt etA AetA.
Note that this is equivalent to injectivity Let T. A linear transformation T from an n dimensional space to itself or an n by n matrix is singular when its determinant vanishes. Thus Tu lies in the.
AB BA I. An n n matrix A is called nonsingular if the only vector x Rn satisfying the equation Ax 0 is x 0. Otherwise the transformation is called singular.
Singular transaction and non singular transactionLinear transformation in hindisingulartransformation nonsingulartransformationlinearalgebra Mathemati. Then A A is nonsingular if and only if the null space of A A is the set containing only the zero vector ie.
Problem Of The Week Find The Nonsingular Matrix Nibcode Solutions

11 Singular And Non Singular Transformation Theorem Based On It Youtube

Singular Transformation And Non Singular Transformation Youtube

Determine Whether The Given 3 By 3 Matrices Are Nonsingular Problems In Mathematics

Non Singular Linear Transformation Definition Theorem Vector Spaces Linear Algebra In Hindi Youtube

Applied Mathematics Fundamental Matrix Linear System How To Apply Matrix

6 Example Of One One Onto Singular Non Singular Linear Transformation For Bsc And Engineering Math Youtube

Rigorously Proving That A Change Of Basis Matrix Is Always Invertible Mathematics Stack Exchange
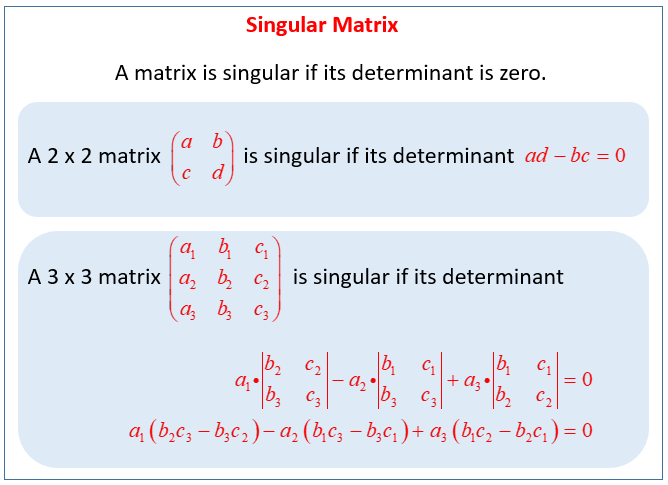
Singular Matrix Video Lessons Examples And Solutions

Solved 2 Let L Be A Linear Transformation Going From R 2 Chegg Com

What Is A Nonsingular Matrix Quora

Singular Matrix And Non Singular Matrix Don T Memorise Youtube

Question Bank Mathematics This Or That Questions Word Search Puzzle

Cramer S Rule Advanced Mathematics Learning Math Logic Math

Non Singular Linear Transformation Definition Theorem Vector Spaces Linear Algebra In Hindi Youtube

Invertible Matrix Definition Deepai

Singular Matrix Explanation Examples

How To Prove A Matrix Is Nonsingular In 10 Seconds Problems In Mathematics
